On this page
Crash Games At Online Casinos
On this page
Introduction
Crash is a simple yet unique type of game. The player sets a winning goal and his probability of achieving it are inversly proportional. The result is displayed to the player as a rocket, increasing in altitude at an exponntial rate. That rocket will eventually explode. The player's aim is to bet on the rocket achieving an altitude equal to the winning goal.
The game can go by other names SmartSoft Gaming calls their version of this game JetX and DraftKings calls it DK Rocket.
The player may set his winning goal to whatever he wishes, up to about 1000x his bet. What happens visually is a space ship takes off at an altitude of 1. Every second, the spaceship either increase in altitude at an exponential rate or explode. The pilot will set a certain altitude to eject. If the pilot ejects at that altitude, before the spaceship explodes, then the player will win the product of his bet and the altitude the pilot ejected.
Rules
The following rules are based on the JetX game by SoftSwiss Gaming.
- The player will choose a bet amount and a winning goal between 1.01 and 1000 times his bet amount.
- After the betting period, which lasts about 5.6 seconds, a spaceship will take off.
- The multiplier (or altitude) of take off is 1.
- About every 1/7 of a second one of two things will happen:
- With about a 99% chance, the multiplier will increase by about 1%.
- With about a 1% chance, the spaceship will explode.
- If the spaceship didn't explode, then if the new multiplier is greater than the player's winning goal, then the pilot will eject and the player will win (on a "for one" basis) his winning goal.
- If the spaceship didn't explode, go back to rule 4.
To make matters more confusing, the player may make one or two bets on different multipliers in the same game. If the player does this, then there will be two pilots in the spacecraft, ejecting at different altitudes as chosen by the player.
The player does not have to bet during the 5.6-second time between games, but may place his next bet during the current game and may also put the game on autoplay to keep repeating the same bets indefinitely.
Example
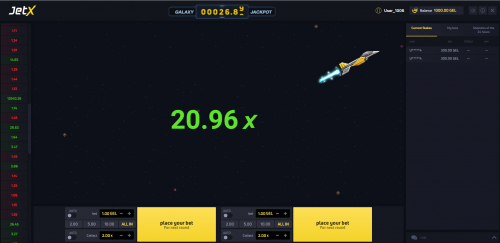
Before making a pair of bets I had a balance of 975 GEL. I have no idea what a GEL is, to be honest. The game was played in fun mode. I made two bets, as shown in the image above. The first bet is shown in the box on the lower left side is for 5 and has a winning goal of 8x my bet, which equals 40. The second bet is shown in the box on the lower right side is for 2 and has a winning goal of 3x my bet, which equals 6. After these bets were confirmed, before this screenshot was taken, my balance is reduced by 7 to 968, as you can see in the balance field at the top.
The second image above was taken a short time after the spaceship reached a multiplier of 3x. As soon as it passed 3x, the first pilot ejected, corresponding to the bet of 2 on a win of 3x. You can't actually see the ejection at first, which is why I waited until 3.16x so you could see the parachute.
The third image above was taken a short time after the spaceship reached a multiplier of 8x. As soon as it passed 8x, the second pilot ejected, corresponding to the bet of 5 on a win of 8x. Again, I waited a few moments to take the screen capture, so you could see the parachute.
Not that it mattered, but the spaceship keeps going up until it explodes, which in this case happened at a multiplier of 11.52x. Also note my balance of 1014 GEL. As a reminder, after making by wagers, my balance was 968. The left side bet returned 5×8 = 40. The right side bet returned 2×3=6. My total win was 40+6=46. My new balance is thus 968+46 = 1014.
Analysis
The return of this game is reported to be 97%.
Given a winning goal of w times the bet amount, the probability of winning is 0.97/w. For example, if the winning goal is 3x the bet amount, then the probability of winning is 0.97/3 = 32.33%. In other words, the probability of winning is inversely proportional to the winning goal.
The way I figure they achieve a 97% return, regardless of the winning goal, is there is 3% chance the spaceship never gets off ground and crashes on the runway. If the spaceship gets off the ground, then the game goes through calculations at a rate of about 400 per minute. At each calculation there is a 99% chance the multiplier grows by 1/99 of its previous value and a 1% chance the spaceship explodes.
The more advanced readers may be interested to know that the average multiplier the spaceship achieves, assuming it gets off the runway, approaches e =~ 2.718281818x as the time until the explosion approaches the exponential distribution.
External Links
Play JetX for fun at the SmartSoft Gaming web site.
Rocket Man!
When I was testing out this game for this article there was a game where the spaceship reached a multiplier of 12540.26. Check the game history on the left side of the image below.